If F(X) Is a Polynomial Then Lim F(X)=f(C) True/false Homework Exercises Review
2. Limits
2.3 The Limit Laws
Learning Objectives
- Recognize the basic limit laws.
- Use the limit laws to evaluate the limit of a function.
- Evaluate the limit of a function by factoring.
- Use the limit laws to evaluate the limit of a polynomial or rational function.
- Evaluate the limit of a role by factoring or by using conjugates.
- Evaluate the limit of a part by using the squeeze theorem.
In the previous section, we evaluated limits by looking at graphs or by constructing a table of values. In this section, we establish laws for calculating limits and larn how to apply these laws. In the Educatee Projection at the finish of this department, you have the opportunity to apply these limit laws to derive the formula for the surface area of a circle by adapting a method devised past the Greek mathematician Archimedes. We begin by restating 2 useful limit results from the previous department. These ii results, together with the limit laws, serve every bit a foundation for computing many limits.
Evaluating Limits with the Limit Laws
The outset two limit laws were stated in (Figure) and we repeat them hither. These basic results, together with the other limit laws, allow us to evaluate limits of many algebraic functions.
Basic Limit Results
For any existent number ![]() and any abiding
and any abiding ![]() ,
,
Evaluating a Basic Limit
Evaluate each of the following limits using (Figure).
We at present take a look at the limit laws, the individual backdrop of limits. The proofs that these laws hold are omitted hither.
We at present do applying these limit laws to evaluate a limit.
Evaluating a Limit Using Limit Laws
Employ the limit laws to evaluate ![]() .
.
Solution
Let'southward apply the limit laws one step at a time to be sure we understand how they work. We need to proceed in mind the requirement that, at each application of a limit police force, the new limits must exist for the limit law to exist applied.

Using Limit Laws Repeatedly
Use the limit laws to evaluate ![]() .
.
Solution
To find this limit, we need to apply the limit laws several times. Over again, nosotros demand to go on in heed that as we rewrite the limit in terms of other limits, each new limit must exist for the limit law to exist applied.

Utilize the limit laws to evaluate ![]() . In each step, indicate the limit law applied.
. In each step, indicate the limit law applied.
Solution
![]()
Limits of Polynomial and Rational Functions
By now y'all accept probably noticed that, in each of the previous examples, it has been the instance that ![]() . This is not e'er true, but information technology does hold for all polynomials for any choice of
. This is not e'er true, but information technology does hold for all polynomials for any choice of ![]() and for all rational functions at all values of
and for all rational functions at all values of ![]() for which the rational function is defined.
for which the rational function is defined.
Limits of Polynomial and Rational Functions
Let ![]() and
and ![]() be polynomial functions. Permit
be polynomial functions. Permit ![]() be a real number. And so,
be a real number. And so,
![]()
![]() .
.
To see that this theorem holds, consider the polynomial ![]() . By applying the sum, constant multiple, and ability laws, nosotros cease up with
. By applying the sum, constant multiple, and ability laws, nosotros cease up with
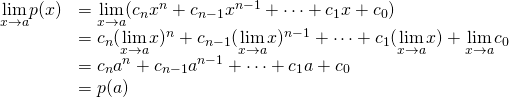
It now follows from the quotient law that if ![]() and
and ![]() are polynomials for which
are polynomials for which ![]() , and then
, and then
![]() .
.
(Figure) applies this effect.
Evaluating a Limit of a Rational Function
Evaluate the ![]() .
.
Solution
Since iii is in the domain of the rational role ![]() , nosotros tin calculate the limit by substituting 3 for
, nosotros tin calculate the limit by substituting 3 for ![]() into the function. Thus,
into the function. Thus,
![]() .
.
Evaluate ![]() .
.
Additional Limit Evaluation Techniques
As we have seen, we may evaluate easily the limits of polynomials and limits of some (only not all) rational functions by direct substitution. Withal, equally nosotros saw in the introductory section on limits, it is certainly possible for ![]() to exist when
to exist when ![]() is undefined. The following observation allows united states to evaluate many limits of this type:
is undefined. The following observation allows united states to evaluate many limits of this type:
If for all ![]() over some open up interval containing
over some open up interval containing ![]() , then
, then ![]() .
.
To understand this thought amend, consider the limit ![]() .
.
The function
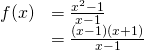
and the function ![]() are identical for all values of
are identical for all values of ![]() The graphs of these two functions are shown in (Figure).
The graphs of these two functions are shown in (Figure).
We see that

The limit has the form ![]() , where
, where ![]() and
and ![]() . (In this case, nosotros say that
. (In this case, nosotros say that ![]() has the indeterminate form 0/0.) The following Trouble-Solving Strategy provides a full general outline for evaluating limits of this type.
has the indeterminate form 0/0.) The following Trouble-Solving Strategy provides a full general outline for evaluating limits of this type.
The next examples demonstrate the employ of this Problem-Solving Strategy. (Figure) illustrates the factor-and-cancel technique; (Figure) shows multiplying by a cohabit. In (Figure), nosotros look at simplifying a complex fraction.
Evaluating a Limit by Factoring and Canceling
Evaluate ![]() .
.
Solution
Step 1. The function ![]() is undefined for
is undefined for ![]() . In fact, if we substitute iii into the office we become 0/0, which is undefined. Factoring and canceling is a skilful strategy:
. In fact, if we substitute iii into the office we become 0/0, which is undefined. Factoring and canceling is a skilful strategy:
![]()
Stride 2. For all ![]() . Therefore,
. Therefore,
![]() .
.
Footstep three. Evaluate using the limit laws:
![]() .
.
Evaluate ![]() .
.
Solution
![]()
Evaluating a Limit by Multiplying by a Conjugate
Evaluate ![]() .
.
Solution
Stride ane. ![]() has the form 0/0 at −1. Let's begin by multiplying by
has the form 0/0 at −1. Let's begin by multiplying by ![]() , the conjugate of
, the conjugate of ![]() , on the numerator and denominator:
, on the numerator and denominator:
![]() .
.
Footstep two. Nosotros then multiply out the numerator. We don't multiply out the denominator considering we are hoping that the ![]() in the denominator cancels out in the end:
in the denominator cancels out in the end:
![]() .
.
Step three. And then we cancel:
![]() .
.
Step 4. Concluding, we utilize the limit laws:
![]() .
.
Evaluate ![]() .
.
Solution
![]()
Evaluating a Limit by Simplifying a Complex Fraction
Evaluate ![]() .
.
Solution
Step 1. ![]() has the form 0/0 at i. Nosotros simplify the algebraic fraction by multiplying past
has the form 0/0 at i. Nosotros simplify the algebraic fraction by multiplying past ![]() :
:
![]() .
.
Pace 2. Next, we multiply through the numerators. Practice not multiply the denominators considering nosotros desire to be able to cancel the factor ![]() :
:
![]() .
.
Pace iii. Then, nosotros simplify the numerator:
![]() .
.
Step four. Now nosotros factor out −one from the numerator:
![]() .
.
Pace 5. Then, we abolish the mutual factors of ![]() :
:
![]() .
.
Pace 6. Last, nosotros evaluate using the limit laws:
![]() .
.
Evaluate ![]() .
.
(Figure) does non fall neatly into any of the patterns established in the previous examples. However, with a little inventiveness, we can still use these same techniques.
Evaluating a Limit When the Limit Laws Do Non Apply
Evaluate ![]() .
.
Solution
Both ![]() and
and ![]() fail to have a limit at zero. Since neither of the two functions has a limit at nada, we cannot apply the sum law for limits; nosotros must utilise a different strategy. In this case, nosotros find the limit by performing addition and and then applying one of our previous strategies. Discover that
fail to have a limit at zero. Since neither of the two functions has a limit at nada, we cannot apply the sum law for limits; nosotros must utilise a different strategy. In this case, nosotros find the limit by performing addition and and then applying one of our previous strategies. Discover that
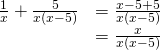
Thus,

Evaluate ![]() .
.
Solution
![]()
Let'due south at present revisit one-sided limits. Simple modifications in the limit laws permit the states to apply them to 1-sided limits. For example, to apply the limit laws to a limit of the class ![]() , we crave the function
, we crave the function ![]() to be divers over an open interval of the form
to be divers over an open interval of the form ![]() ; for a limit of the form
; for a limit of the form ![]() , we require the function
, we require the function ![]() to be defined over an open interval of the form
to be defined over an open interval of the form ![]() . (Figure) illustrates this point.
. (Figure) illustrates this point.
Evaluating a 1-Sided Limit Using the Limit Laws
Evaluate each of the following limits, if possible.
In (Figure) we look at one-sided limits of a piecewise-defined function and use these limits to depict a conclusion nigh a ii-sided limit of the same function.
Evaluating a Two-Sided Limit Using the Limit Laws
Graph 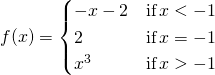 and evaluate
and evaluate ![]() .
.
Solution
 -1, which crossed the x axis and y centrality at the origin.">
-1, which crossed the x axis and y centrality at the origin.">
![]()
We at present plough our attending to evaluating a limit of the grade ![]() , where
, where ![]() , where
, where ![]() and
and ![]() . That is,
. That is, ![]() has the form
has the form ![]() at
at ![]() .
.
Evaluating a Limit of the Form  Using the Limit Laws
Using the Limit Laws
Evaluate ![]() .
.
Evaluate ![]() .
.
Solution
![]()
The Squeeze Theorem
The techniques we have developed thus far work very well for algebraic functions, but we are still unable to evaluate limits of very basic trigonometric functions. The next theorem, called the squeeze theorem, proves very useful for establishing basic trigonometric limits. This theorem allows u.s. to calculate limits by "squeezing" a function, with a limit at a point ![]() that is unknown, between two functions having a common known limit at
that is unknown, between two functions having a common known limit at ![]() . (Figure) illustrates this idea.
. (Figure) illustrates this idea.
The Squeeze Theorem
Let ![]() , and
, and ![]() be defined for all
be defined for all ![]() over an open interval containing
over an open interval containing ![]() . If
. If
![]()
for all ![]() in an open interval containing
in an open interval containing ![]() and
and
![]()
where ![]() is a real number, then
is a real number, then ![]() .
.
Applying the Squeeze Theorem
Utilize the Squeeze Theorem to evaluate ![]() .
.
Use the Squeeze Theorem to evaluate ![]() .
.
We now use the Clasp Theorem to tackle several very important limits. Although this give-and-take is somewhat lengthy, these limits show invaluable for the development of the material in both the next section and the side by side chapter. The beginning of these limits is ![]() . Consider the unit circle shown in (Figure). In the figure, we come across that
. Consider the unit circle shown in (Figure). In the figure, we come across that ![]() is the
is the ![]() -coordinate on the unit circle and it corresponds to the line segment shown in blueish. The radian measure of angle θ is the length of the arc it subtends on the unit circle. Therefore, we see that for
-coordinate on the unit circle and it corresponds to the line segment shown in blueish. The radian measure of angle θ is the length of the arc it subtends on the unit circle. Therefore, we see that for ![]() .
.

Considering ![]() and
and ![]() , by using the Clasp Theorem we conclude that
, by using the Clasp Theorem we conclude that
![]() .
.
To see that ![]() likewise, observe that for
likewise, observe that for ![]() and hence,
and hence, ![]() . Consequently,
. Consequently, ![]() It follows that
It follows that ![]() . An application of the Squeeze Theorem produces the desired limit. Thus, since
. An application of the Squeeze Theorem produces the desired limit. Thus, since ![]() and
and ![]() ,
,
![]() .
.
Next, using the identity ![]() for
for ![]() , we see that
, we see that
![]() .
.
Nosotros at present take a wait at a limit that plays an of import function in later chapters—namely, ![]() . To evaluate this limit, we use the unit of measurement circle in (Figure). Detect that this effigy adds i additional triangle to (Figure). Nosotros see that the length of the side opposite angle
. To evaluate this limit, we use the unit of measurement circle in (Figure). Detect that this effigy adds i additional triangle to (Figure). Nosotros see that the length of the side opposite angle ![]() in this new triangle is
in this new triangle is ![]() . Thus, we run into that for
. Thus, we run into that for ![]() .
.

Past dividing by ![]() in all parts of the inequality, nosotros obtain
in all parts of the inequality, nosotros obtain
![]() .
.
Equivalently, we have
![]() .
.
Since ![]() , we conclude that
, we conclude that ![]() . By applying a manipulation similar to that used in demonstrating that
. By applying a manipulation similar to that used in demonstrating that ![]() , we can prove that
, we can prove that ![]() . Thus,
. Thus,
![]() .
.
In (Effigy) we use this limit to establish ![]() . This limit too proves useful in later capacity.
. This limit too proves useful in later capacity.
Evaluating an Important Trigonometric Limit
Evaluate ![]() .
.
Solution
In the starting time pace, we multiply by the conjugate so that we can use a trigonometric identity to convert the cosine in the numerator to a sine:
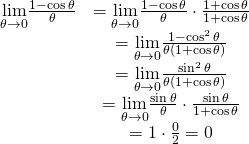
Therefore,
![]() .
.
Evaluate ![]() .
.
Key Concepts
- The limit laws permit us to evaluate limits of functions without having to go through step-by-pace processes each time.
- For polynomials and rational functions,
 .
. - Yous can evaluate the limit of a role by factoring and canceling, by multiplying by a conjugate, or past simplifying a complex fraction.
- The Squeeze Theorem allows you lot to detect the limit of a function if the part is always greater than one function and less than another function with limits that are known.
Primal Equations
In the post-obit exercises, use the limit laws to evaluate each limit. Justify each step by indicating the advisable limit law(s).
i. ![]()
Solution
Utilise abiding multiple police and difference law: ![]()
two. ![]()
iii. ![]()
Solution
Use root law: ![]()
4. ![]()
In the post-obit exercises, utilise direct substitution to evaluate each limit.
5. ![]()
six. ![]()
seven. ![]()
eight. ![]()
nine. ![]()
Solution
![]()
10. ![]()
In the following exercises, use direct substitution to show that each limit leads to the indeterminate form 0/0. Then, evaluate the limit.
12. ![]()
14. ![]()
xvi. ![]() , where
, where ![]() is a existent-valued abiding
is a existent-valued abiding
xviii. ![]()
20. ![]()
In the following exercises, apply direct substitution to obtain an undefined expression. Then, use the method of (Figure) to simplify the role to aid determine the limit.
21. ![]()
Solution
![]()
22. ![]()
23. ![]()
Solution
![]()
24. ![]()
In the following exercises, assume that ![]() , and
, and ![]() . Use these three facts and the limit laws to evaluate each limit.
. Use these three facts and the limit laws to evaluate each limit.
25. ![]()
Solution
![]()
26. ![]()
27. ![]()
Solution
![]()
28. ![]()
29. ![]()
Solution
![]()
xxx. ![]()
31. ![]()
Solution
![]() .
.
32. ![]()
In the following exercises, utilize a calculator to describe the graph of each piecewise-divers function and study the graph to evaluate the given limits.
Solution
 iii. There is an open circle at (3, seven), and the slope is ane.">
iii. There is an open circle at (3, seven), and the slope is ane.">
a. 9; b. vii
Solution
 = 2. It has a slope of -1 and an x intercept at (three,0).">
= 2. It has a slope of -1 and an x intercept at (three,0).">
a. one; b. 1
In the following exercises, employ the following graphs and the limit laws to evaluate each limit.
 -3. Other central points are (0, 1), (-v,2), (i,2), (-7, 4), and (-nine,6). The lower piecewise function has a linear segment and a curved segment. The linear segment exists for x < -3 and has decreasing gradient. Information technology goes to (-3,-two) at x=-3. The curved segment appears to be the right half of a downward opening parabola. It goes to the vertex point (-3,2) at x=-3. It crosses the y axis a piddling below y=-2. Other key points are (0, -vii/three), (-five,0), (1,-5), (-7, 2), and (-9, 4).">
-3. Other central points are (0, 1), (-v,2), (i,2), (-7, 4), and (-nine,6). The lower piecewise function has a linear segment and a curved segment. The linear segment exists for x < -3 and has decreasing gradient. Information technology goes to (-3,-two) at x=-3. The curved segment appears to be the right half of a downward opening parabola. It goes to the vertex point (-3,2) at x=-3. It crosses the y axis a piddling below y=-2. Other key points are (0, -vii/three), (-five,0), (1,-5), (-7, 2), and (-9, 4).">
36. ![]()
37. ![]()
Solution
![]()
38. ![]()
39. ![]()
Solution
![]()
40. ![]()
41. ![]()
[reveal-respond q="957757″]Show Solution[/reveal-answer]
[hidden-answer a="957757″]
![]()
42. ![]()
43. ![]()
Solution
![]()
For the following problems, evaluate the limit using the Clasp Theorem. Use a calculator to graph the functions ![]() , and
, and ![]() when possible.
when possible.
44. [T] True or Simulated? If ![]() , then
, then ![]() .
.
45. [T] ![]()
Solution
The limit is zero.
![The graph of three functions over the domain [-1,1], colored red, green, and blue as follows: red: theta^2, green: theta^2 * cos (1/theta), and blue: - (theta^2). The red and blue functions open upwards and downwards respectively as parabolas with vertices at the origin. The green function is trapped between the two.](https://s3-us-west-2.amazonaws.com/courses-images/wp-content/uploads/sites/2332/2018/01/11203457/CNX_Calc_Figure_02_03_206.jpg)
46. ![]() , where
, where 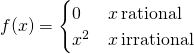
Solution
a.

b. ![]() . The magnitude of the electric field as yous arroyo the particle
. The magnitude of the electric field as yous arroyo the particle ![]() becomes space. It does non make physical sense to evaluate negative distance.
becomes space. It does non make physical sense to evaluate negative distance.
Source: https://opentextbc.ca/calculusv1openstax/chapter/the-limit-laws/
0 Response to "If F(X) Is a Polynomial Then Lim F(X)=f(C) True/false Homework Exercises Review"
Post a Comment